Minor chord shapes on guitar (CAGED minor chords)

As has been discussed in the previous sections on the CAGED system, the basic major shapes (the C,A,G,E and D major shapes) lend themselves to production of all other major chords. From that we have seen that there is a more general principle to chord structure formation.

Now referring to the section "Composition and Naming of Chords" at the beginning of chapter 6, we can get a comprehensive list of which notes make up which kind of chord (e.g. major chord = root note (R) + major 3rd (M3) + perfect 5th (P5)). This list will be referred to on several occasions in the following section.

Having gone through all that, we can now proceed to understand minor chord shapes:

All minor chords are composed of: a root note (R) + minor 3rd (m3) + perfect 5th (P5) notes. Hence, to get from major chords to minor chords, we must simply replace the major 3rd (M3) notes with minor 3rd (m3) notes. Recalling from the section on musical intervals, we see that the major third is a semitone away from the minor 3rd note, counting from unison. Thus, to produce minor chords, all major 3rd notes being played within the shapes must be reduced by a semitone (shifted back by one fret) while keeping the root and P5 notes in place. For example:

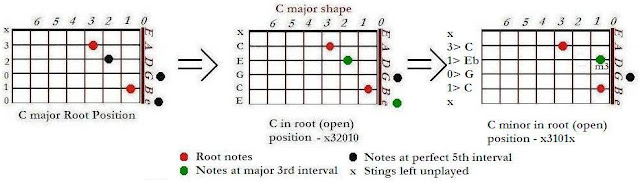
1. The C major shape can be transformed to a C minor (Cm) shape like so:

In the C major chord, the major 3rd notes (E) were on the 4th string, 2nd fret and on the 1st string in open. To convert this to a minor chord, we changed the major 3rd note (E) on the 4th string, 2nd fret, to a minor 3rd note (Eb) played on the 4th string, 1st fret. The other note, being on the a sting in open position, could not be pushed back further. Thus, that string was excluded from the new minor shape. This C minor shape is not common, but it comes straight from theory.

This shape (C minor (Cm) in open position, played as x3101x) is also, now movable, producing C#m at x4212x, Dm at x5323x, Ebm / D#m at x6434x, and so on.

2. Again, A minor (Am) in open position can be similarly derived from the A major shape:

Here, again, we convert the major 3rd on the 2nd string, second fret to a minor 3rd on the 2nd string, 1st fret producing the A minor shape, x02210. As above, this shape too is movable producing A#m / Bbm at x13321, Bm at x24432, Cm at x35543, C#m at x46654 and so on. This is one of the common minor shapes.

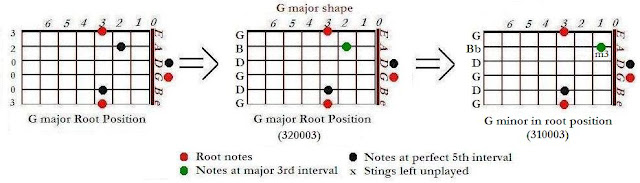
3. Modifying the G major shape, we get a very uncommon G minor (Gm) shape:

Shifting this shape down produces similarly uncommon variants of G#m at 421114, Am at 532225, etc.

4. Modifying the E major shape produces the familiar E minor (Em) chord shape:

Shifting this shape of E minor (022000) gives us several common minor chord shapes, viz. Fm at 133111, F#m at 244222, Gm at 344333, G#m at 466444, Am at 577555, and so on.

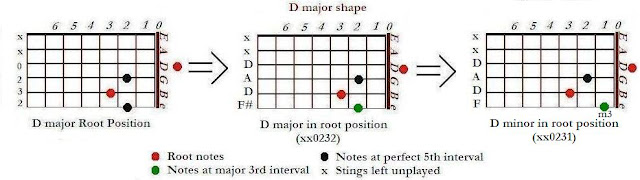
5. Finally, modifying the D major shape, we get the common D minor (Dm) chord shape:

This shape of Dm (xx0231), when shifted down, produces Ebm / D#m at xx1342, Em at xx2453, Fm at xx3564, and so on. This is also a common minor form. This shape is often modified as xx0231 > xx3231, but this is the 1st inversion of this minor shape.

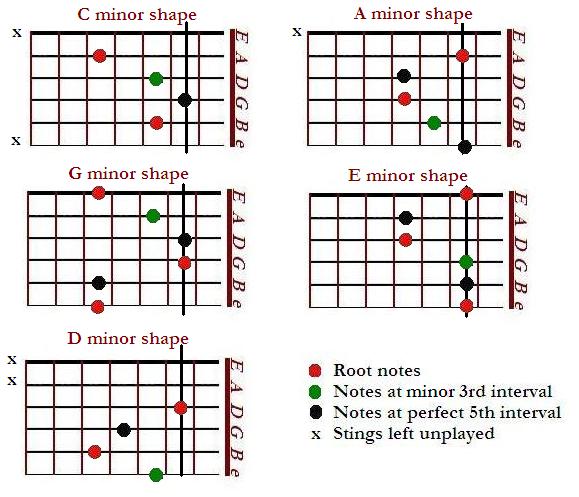
Finally, let us have a look at the derived movable (with bar) minor chord shapes all together:

The CAGED System on a Guitar:

• Introduction to the CAGED system
• Fretboard layout
• Bar chords and movable shapes
• The basic 5 CAGED shapes
• CAGED Major chords: C Major, A Major, G Major, E Major, D Major
• Non-CAGED Major chords : F major, B major, C#, Eb, etc
• Principle of CAGED system
• CAGED Minor chords
• Fretboard note map
• Extended guitar chords

http://basicmusictheory.blogspot.com/2010/08/minor-chord-shapes-on-guitar-caged.html

Liked 'Minor chord shapes on guitar (CAGED minor chords)' enough to share / save?

Minor chord shapes on guitar (CAGED minor chords)